Tyndall Effect, Scientifically Explained

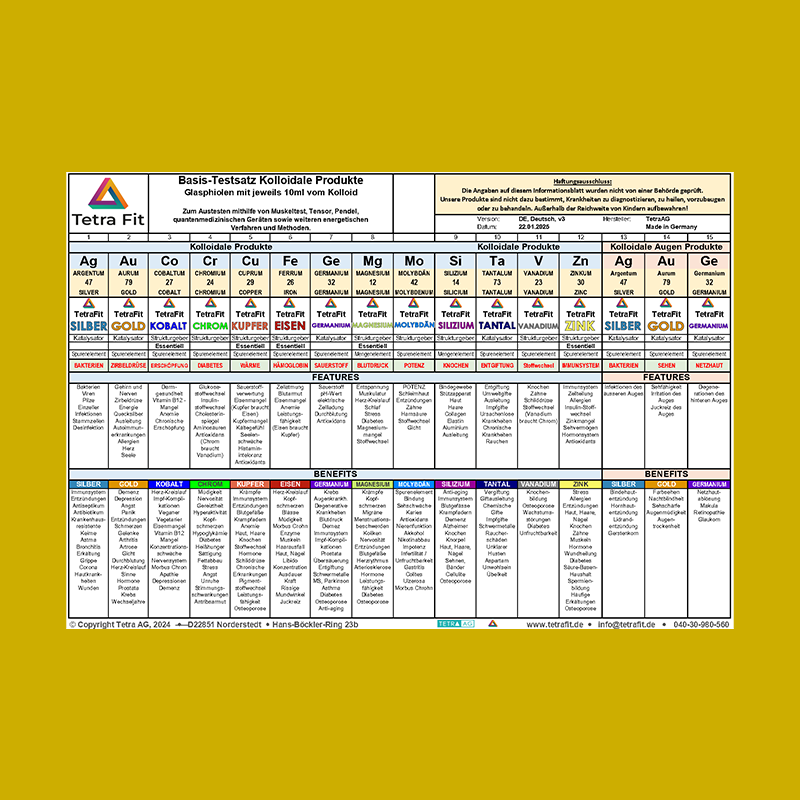
To detect the tiny nanoparticles in a colloidal solution, the aqueous solution is often illuminated with a laser beam. If the laser beam is visible, this can indicate the presence of a colloidal solution containing nanoparticles.

The Tyndall effect describes the scattering of light by particles in a colloidal solution or suspension. This causes the path of light to become visible as the light is scattered. The effect is a consequence of Rayleigh scattering for particles that are larger than air molecules but smaller than the wavelength of light.

1. Basic equation of light scattering (Rayleigh scattering)

The intensity of the scattered light $I$ depends on the wavelength $\lambda$ of the light and the particle diameter $d$ . The Rayleigh scattering is given by:

$$I \propto \frac{I_0}{\lambda^4} d^6$$

where:

• $I_0$ = intensity of the incident light,
• $\lambda$ = wavelength of light,
• $d$ = diameter of the particles.

Since the scattering intensity is inversely proportional to $\lambda^4$ , blue light is scattered more than red light, which is why the Tyndall effect often appears as bluish scattering.

---

2. Mie scattering for larger particles

For larger particles ( $d > 0.1\lambda$ ) the Mie theory applies, which provides a more precise description. The scattering is then no longer strongly wavelength-dependent, which is why white light is scattered. The scattering can be calculated using the Mie solution of the Maxwell equations:

$$Q_s = \frac{2}{x^2} \sum_{n=1}^{\infty} (2n + 1) (|a_n|^2 + |b_n|^2)$$

with:

• $Q_s$ = scattering cross section,
• $x = \frac{2\pi d}{\lambda}$ = dimensionless particle size,
• $a_n, b_n$ = Mie coefficients, which depend on particle size and refractive index.

For $x \approx 1$ (particle size in the range of the wavelength of light) the Tyndall effect occurs.

---

3. Visibility of the Tyndall effect

The perceptibility of the Tyndall effect depends on the ratio of the particle diameter to the wavelength of the light. Typically, the effect occurs when:

$$0.01\lambda < d < 10\lambda$$

This means that particles with a diameter between about 10 nm and 10 µm show the Tyndall effect.

---

4. Application of extinction legislation

The attenuation of light through scattering can be described by the Lambert-Beer law :

$$I = I_0 e^{-\alpha x}$$

with:

• $\alpha$ = extinction coefficient (depending on particle size and concentration),
• $x$ = optical path length.

For colloidal solutions, the scattering can be further refined by the Kubelka-Munk theory :

$$R = \frac{(1 - \sqrt{1 - 2K})}{(1 + \sqrt{1 - 2K})}$$

Summary:

• Rayleigh scattering dominates for small particles and leads to bluish scattering.
• The Mie theory describes scattering for larger particles and can lead to a whitish appearance.
• The Tyndall effect occurs when the particle size is between 10 nm and 10 µm .
• The intensity of the scattering is inversely proportional to the fourth power of the wavelength ( $1/\lambda^4$ ), whereby shorter wavelengths (blue) are scattered more strongly than longer ones (red).